Algebra is the branch of Math which uses alphabetical letters to find unknown numbers. These letters are also called variables. The values are known in the given expression such as numbers are called constants. When we speak about its basics, it covers the general algebraic expressions, formulas and identities, which are used to solve many mathematical problems.
Mathematics like addition, subtraction, multiplication, and division involve both constants as well as variables. For example, x + 10 = 0. This introduces an important algebraic concept known as equations. The algebraic equation can be thought of as a scale where the weights are balanced through numbers or constants.
Algebra Basics
Algebra is the branch of mathematics that helps in the representation of problems or situations in the form of mathematical expressions. It involves variables like x, y, z, and mathematical operations like addition, subtraction, multiplication, and division to form a meaningful mathematical expression. All the branches of mathematics such as trigonometry, calculus, and coordinate geometry, involve the use of algebra. One simple example of an expression in algebra is 2x + 4 = 8.
Algebra Rules
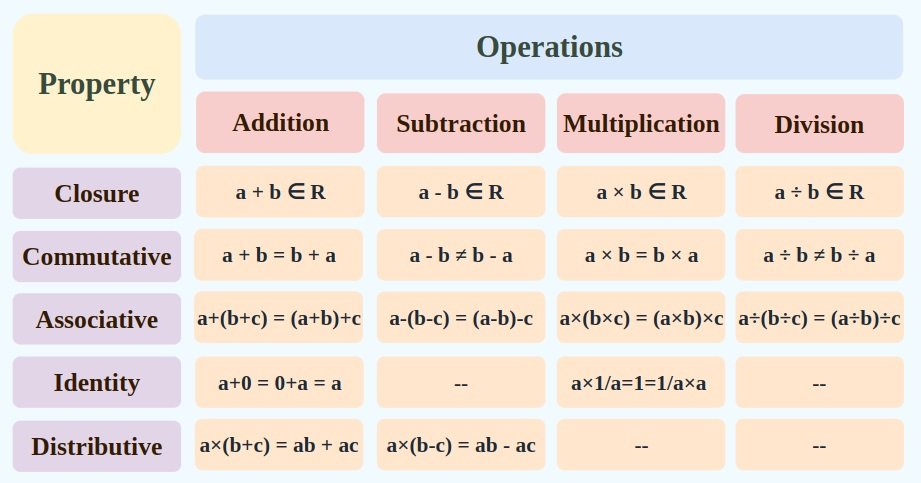
There are five basic rules of algebra including:
- Commutative Rule of Addition
- Commutative Rule of Multiplication
- Associative Rule of Addition
- Associative Rule of Multiplication
- Distributive Rule of Multiplication
These rules are explained in the image below:
Algebraic Operations
The four basic algebraic operations are:
- Addition
- Subtraction
- Multiplication
- Division
Algebraic Expressions
An algebraic expression in algebra is formed using integer constants, variables, and basic arithmetic operations of addition(+), subtraction(-), multiplication(×), and division(/). An example of an algebraic expression is 5x + 6. Here 5 and 6 are fixed numbers and x is a variable. Further, the variables can be simple variables using alphabets like x, y, z or can have complex variables like x2, x3, xn, xy, x2y, etc.
Algebraic expressions are also known as polynomials. A polynomial is an expression consisting of variables (also called indeterminates), coefficients, and non-negative integer exponents of variables.
Example: 6x3 + 4x2 + 5x + 2 = 0
Linear Equations
Linear equations help in representing the relationship between variables such as x, y, z, and are expressed in exponents of one degree. In these linear equations, we use algebra, starting from the basics such as the addition and subtraction of algebraic expressions.
Quadratic Equations
A quadratic equation can be written in the standard form as ax2 + bx + c = 0, where a, b, c are constants and x is the variable. The values of x that satisfy the equation are called solutions of the equation, and a quadratic equation has at most two solutions.
Cubic Equations
Algebraic equations having variables with power 3 are referred to as cubic equations. A generalized form of a cubic equation is ax3 + bx2 + cx + d = 0. A cubic equation has numerous applications in calculus and three-dimensional geometry (3D Geometry).
Sequence and Series
A set of numbers having a relationship across the numbers is called a sequence. A sequence is a set of numbers having a common mathematical relationship between the number, and a series is the sum of the terms of a sequence. In mathematics, we have two broad number sequences and series in the form of arithmetic progression and geometric progression. Some of these series are finite and some series are infinite.
The two series are also called arithmetic progression and geometric progression and can be represented as follows.
Arithmetic Progression
Arithmetic progression (AP) is a special type of progression in which the difference between two consecutive terms is always a constant. The terms of an arithmetic progression series is a, a+d, a + 2d, a + 3d, a + 4d, a + 5d, …
Geometric Progression
Any progression in which the ratio of adjacent terms is fixed is a Geometric Progression. The general form of representation of a geometric sequence is a, ar, ar2, ar3, ar4, ar5, …
Basic Algebra Examples
Example 1: Find y, when, y + 15 = 20
Solution:
y + 15 = 20
y = 20 – 15
y = 5
Example 2: Find x, when, 5x = 65
Solution:
5x = 65
x = 65/5
x = 13
Example 3: If x/7 = 7, then find x.
Solution:
Given,
x/7 = 7
x = 7 × 7
x = 49
Example 4: Solve the equation 5x – 6 = 3x – 8.
Solution:
Given,
5x – 6 = 3x – 8
Adding 6 on both sides,
5x – 6 + 6 = 3x – 8 + 6
5x = 3x – 2
Subtract 3x from both sides,
5x – 3x = 3x – 2 – 3x
2x = -2
Dividing both sides of the equation by 2,
2x/2 = -2/2
x = -1
Example 5: Solve 2ex + 5 = 115
Solution:
Given,
2ex + 5 = 115
2ex = 115 – 5 = 110
ex = 110/2 = 55
x = ln 55
FAQs on Algebra
What are the Branches of Algebra?
Branches of Algebra are:
- Advanced Algebra
- Elementary Algebra
- Abstract Algebra
- Linear Algebra
- Commutative Algebra
What are Types of Algebraic Equations?
Five main types of algebraic equations are:
- Monomial or Polynomial Equations
- Exponential Equations
- Trigonometric Equations
- Logarithmic Equations
- Rational Equations
What is Algebra?
Algebra is a branch of mathematics that deals with solving equations and finding the values of variables. It can be used in different fields such as physics, chemistry, and economics to solve problems. Algebra is not just solving equations but also understanding the relationship between numbers, operations, and variables.
What are the 5 Basic Rules of Algebra?
Basic rules of algebra are:
- Commutative Rule of Addition
- Commutative Rule of Multiplication
- Associative Rule of Addition
- Associative Rule of Multiplication
- Distributive Rule of Multiplication
What is the Golden Rule of Algebra?
Golden Rule of Algebra states that, “Do unto one side of the equal sign as you will do to the other…Whatever you do on one side of the equal sign, you MUST do the same exact thing on the other side.”




