In mathematics, a percentage is a figure or ratio that signifies a fraction out of 100 i.e., A fraction whose denominator is 100 is called a Percent i.e. in all the fractions where the denominator is 100 we can remove the denominator and put the % sign.
For example, the fraction 23/100 can be written as 23%. The opposite of this is also true i.e. any percentage sign can be easily replaced by converting the number into a fraction with the denominator 100. For example, 45% can be converted to a fraction as 45/100.
The word “Percentage” was coined from the Latin word “Percentum” which means “by hundred“. It is a dimensionless relation between two numbers. It is often denoted by the sign “%” or percent or pct.
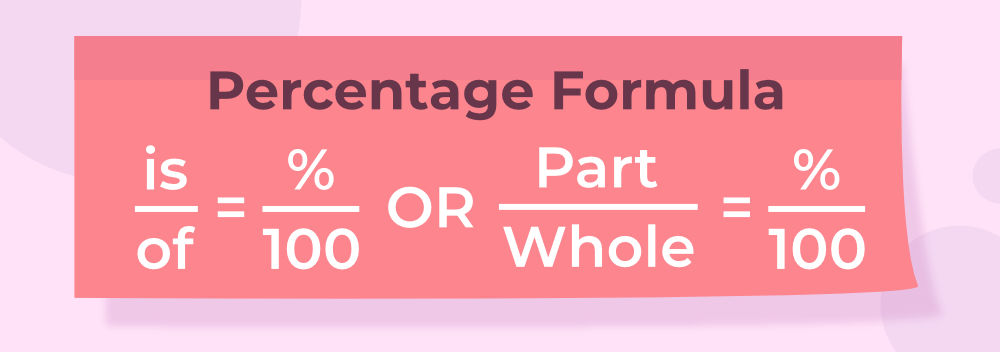
Percentage Formula
Percentage formula is a formula that is used to find the amount or share of a quantity in terms of a hundred. So, for calculating the percentage, we basically need three variables. First, the total value V1, the present value V2, and the percentage value P. The algebraic equation for this will be:
Percentage (P%) = (Parts (V2) / Whole (V1)) × 100
How to Calculate Percentage of a Number?
Calculating percentage of a number is very simple, you just need to use the formula mentioned below:
Percent of a Number = Percentage/100 × Number
Example: Calculate 5% of 50
- 5% of 50 = 5/100 × 50
- 5% of 50 = 0.05 × 50
- 5% of 50 = 2.50
Percentage Difference Formula
Percentage difference or the percentage change formula is calculated when the difference between two values is divided by the average of the same values. We can say that the percentage difference is used to calculate the change in the value over the given period. Mathematically, we can be written as,
Percentage Difference = (Absolute difference / Average) × 100
Example: Percentage difference between 50 and 100 will be:
= |50-100|/ {(50+100)/2} × 100
= 50/75 ×100
= 66.66%
Note: The image given below shows us some examples where the percentage is used.

Percentage Chart
Let’s see the percentage chart of fractions converted into percentages,
|
Percentage Chart |
|||
|---|---|---|---|
| Fraction | Percentage | Fraction | Percentage |
| 1/1 | 100% | 1/11 | 9.09% |
| 1/2 | 50% | 1/12 | 8.33% |
| 1/3 | 33.33% | 1/13 | 7.69% |
| 1/4 | 25% | 1/14 | 7.14% |
| 1/5 | 20% | 1/15 | 6.66% |
| 1/6 | 16.66% | 1/16 | 6.25% |
| 1/7 | 14.28% | 1/17 | 5.88% |
| 1/8 | 12.5% | 1/18 | 5.55% |
| 1/9 | 11.11% | 1/19 | 5.26% |
| 1/10 | 10% | 1/20 | 5% |
Percentage Tricks
There are percentage tricks that can be used while calculating the percentage of numbers. Below given trick is the most used,
% x of y = % y of x
Example: Solve 300% of 50.
Solution:
Here, solving 300% of 50 can be a little lengthy and tricky. However, using the trick it can be easily solved,
%x of y = %y of x
300% of 50 = 50% of 300
Now, solving 50% of 300 is relatively is. 50% of 300 is just half of 300. Therefore, 50% of 300 is 150.
Therefore, 300% of 50 is 150.
Solved Example of Percentage
Some examples on the percentages are,
Example 1: Find 15 % of 500.
Solution:
We can find the percentage by formula,
V2 = P × V1
⇒ V2 = 15% × 500
⇒ V2 = (15 × 500) / 100
⇒ V2 = 75.Thus, 15% of 500 is 75.
Example 2: What percentage is 1 of 3000?
Solution:
We can find the percentage by formula,
V2 = P × V1
⇒ P = V2 / V1
⇒ P = 1 / 3000Thus, P% = 1/3000 × 100
⇒ P% = (1/30)%Thus, (1/30)% of 2000 is 500.
Example 4: If 10% of x is 900. Find x.
Solution:
We can find the percentage by the formula,
V2 = P × V1
⇒ V1 = V2 / P
⇒ V1 = (V2 × 100 ) / P%
⇒ V1 = (900 × 100) / 10
⇒ V1 = 9000Thus, the value of x is 9000.
Example 5: Find the value of the percentage of green blocks in each case.
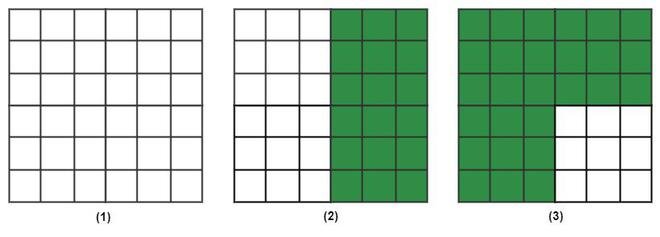
Solution:
In first case, the green blocks are 0, and the total blocks are 36.
Therefore,Percentage of green Blocks = 0/36 × 100 = 0%.
In second case, the green blocks are 18, and the total blocks are 36.Therefore, Percentage of green Blocks = 18/36 × 100 = 50%.
In third case, the green blocks are 27 and the total bricks are 36.Therefore, Percentage of green Blocks = 27/36 × 100 = 75%.




